Multiparameter processes
In order to use stochastic processes to represent the variability of multidimensional phenomena, it is necessary to define extensions for indices in R^N (N ≥ 2) . For instance, two different kinds of extensions of multifractional Brownian motion have been considered: an isotropic extension using the Euclidean norm of R^N and a tensor product of one-dimensional processes on each axis.
These works [1] have highlighted the difficulty of giving satisfactory definitions for increment stationarity, Hölder continuity and covariance structure which are not closely dependent on the structure of R^N.
For example, the Euclidean structure can be unadapted to represent natural phenomena.
A promising improvement in the definition of multiparameter extensions is the concept of set-indexed processes. A set-indexed process is a process whose indices are no longer “times” or “locations” but may be some compact connected subsets of a metric measure space.
Set-indexed processes allow for greater flexibility, and should in particular be useful for the modelling of censored data. This situation occurs frequently in biology and medicine.
A set-indexed extension of fBm is the first step toward the modelling of irregular phenomena within this more general frame. The so-called set-indexed fractional Brownian motion (sifBm) [ref] is defined as the mean-zero Gaussian process {BH,U ; U ∈ A} such that:
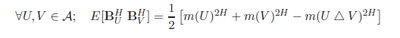
where A is a collection of connected compact subsets of a measure metric space and 0 < H ≤1/2.
This process appears to be the only set-indexed process whose projection on increasing paths is a one-parameter fractional Brownian motion [2]. The construction also provides a way to define fBm’s extensions on non-euclidean spaces, e.g. indices can belong to the unit hyper-sphere of RN. The study of fractal properties needs specific definitions for increment stationarity and self-similarity of set-indexed processes [ref]. We have proved that the sifBm is the only Gaussian set-indexed process satisfying these two (extended) properties [2].
The increment stationarity property for set-indexed processes, previously defined in the study of the sifBm, allows to consider set-indexed processes whose increments are independent and stationary. This generalizes the definition of Bass-Pyke and Adler-Feigin for Lévy processes indexed by subsets of RN, for a more general indexing collection. We have obtained a Lévy-Khintchine representation for these set-indexed Lévy processes and we also characterized this class of Markov processes [3].
In [4], a Kolmogorov regularity theorem is presented, and leads to the definition of various exponents of Hölder regularity for set-indexed processes. For instance, some results concern the regularity exponents of the incremental process, and the exponent of pointwise continuity. Pointwise continuity is defined in [3] and is to be linked to the regularity of set-indexed Lévy processes. When the indexing collection is not "too rich", Gaussian processes are proved to have Hölder continuous sample paths almost surely. In particular, the local and pointwise Hölder exponents of a sifBm are a.s. uniformly equal to the Hurst parameter H.
Our current work in this area focuses on:
- further theoretical studies of the set-indexed fractional Brownian motion, in particular, the local regularity of its sample paths.
- For Lévy processes, continuity and regularity properties appear more complicated to describe in the set-indexed frame than in the real-parameter case. For instance, the absence of total ordering
prevents from characterizing discontinuities with jump instants and size of jumps. - Defining and studying a set-indexed Gaussian process whose regularity would vary along its sample paths.
References:
[4] E. Herbin, A. Richard. Local Hölder regularity of set-indexed processes. Submitted, 2012.
Paul R. Halmos - Lester R. Ford Award for for the article "Christiane's Hair" by J. Lévy Véhel and F. Mendivil
