Multifractional brownian motion
One of the simplest stochastic process for which some kind of control over the Hölder exponents is possible is probably fractional Brownian motion (fBm). One definition of fBm reads as follows:
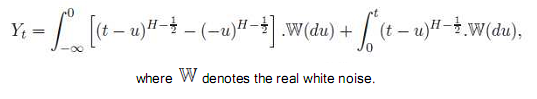
The parameter H ranges in (0, 1), and it governs the pointwise regularity: indeed, almost surely, at each point, both the local and pointwise Hölder exponents are equal to H.
Although varying H yields processes with different regularity, the fact that the exponents are constant along any single path is often a major drawback for the modelling of real world phenomena. For instance, fBm has often been used for the synthesis natural terrains. This is not satisfactory since it yields images lacking crucial features of real mountains, where some parts are smoother than others, due, for instance, to erosion.
It is possible to generalize fBm to obtain a Gaussian process for which the pointwise Hölder exponent may be tuned at each point: multifractional Brownian motion (mBm) is such an extension, obtained by substituting the constant parameter H ∈ (0, 1) with a regularity function H ranging in (0, 1):

The Hölder exponents of mBm are prescribed almost surely: almost surely, the pointwise (resp. local) Hölder exponent at t is the minimum between H(t) and the pointwise (resp. local) exponent of H at t.
Multifractional Brownian motion is our prime example of a stochastic process with prescribed local regularity.
The fact that the local regularity of mBm may be tuned via a functional parameter has made it a useful model in various areas such as finance, biomedicine, geophysics, image analysis. . . A large number of studies have been devoted worldwide to its mathematical properties, e.g. its local time. In addition, there is now a rather strong body of work dealing the estimation of its functional parameter. See here for a partial list of works that use mBm.
Click below for a short animation showing mBms with various h functions:
Left: H function (green) and its GQV estimation (red). Right: corresponding mBm. Animation O. Barrière.
We study the following points in relation with mBm:
- Neither fractional Brownian motion nor multifractional Brownian motion are semi-martingales. Therefore, the question of stochastic integration requires specific developments. There exists a large amount of recent work about stochastic integration with respect to fBm, or, more generally, Gaussian processes. We are studying extensions or specializations of these works to the particular case of mBm.
One possibility is to define the integral with respect to mBm as a limit of integrals with respect to fBm, by approximating mBm by tangents fBms. Another one is to use Wick-Ito integrals. - Although a number of estimators have been proposed for evaluating the function H, some of which yielding accurate results in one dimension, there is still room for progress, in particular in higher dimensions. We are particularly interested in the two-dimensional case in the frame of our application to natural terrains modeling. In addition, one usually observes G(t)X(t) rather that X(t), where G(t) is a smooth deterministic function. Estimating G still poses some difficulties.